Yax2+bx
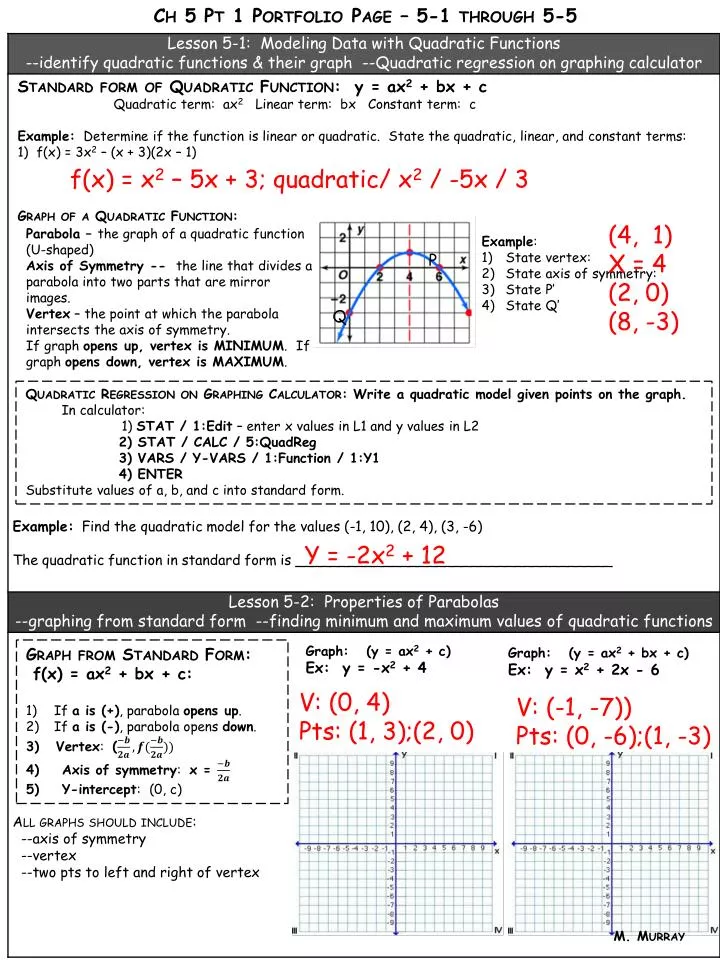
Given y = ax 2 + bx + c , we have to go through the following steps to find the points and shape of any parabola:.

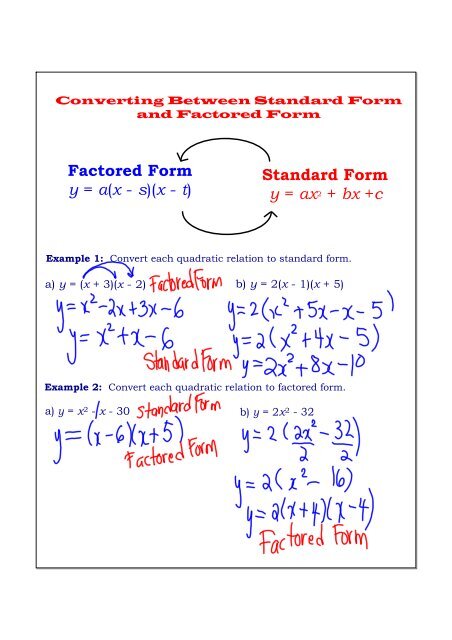
Yax2+bx. Aksed find the equation oft he parabola y=ax^2 + bx +c. The graph is a parabola and hence has an equation y = k(x - v)^2 + h, where (v,h) are the coordinates of the vertex. If the parabola intersects the x -axis in two points, there are two real roots, which are the x -coordinates of these two points (also called x -intercept).
Move all terms containing a to the left, all other terms to the right. In the standard form, y = ax 2 + bx + c, a parabolic equation resembles a classic quadratic equation. Graph y = 2x2 - 4.
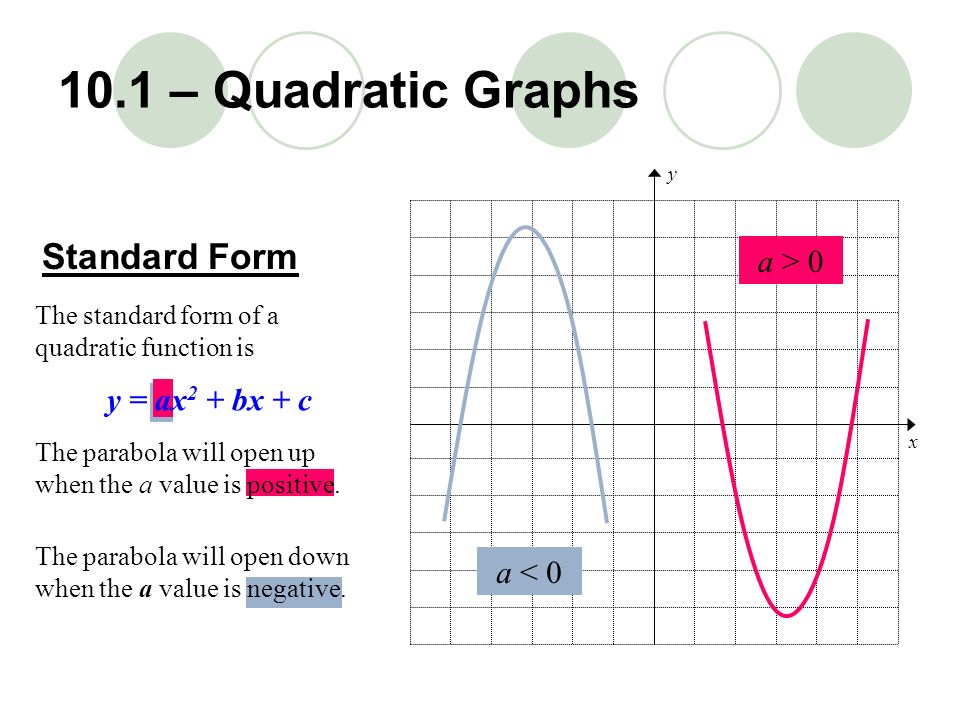
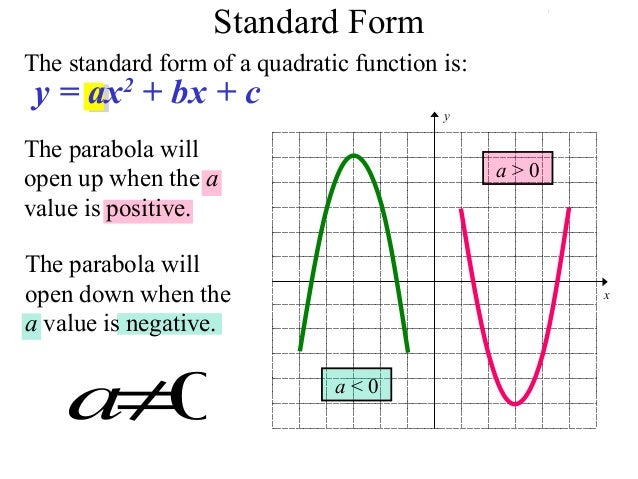
Ax + by + c + x 2 + -1by + y 2 = 0 + -1by Reorder the terms:. -√b is the negative square root. If a > 0 (positive) then the parabola opens upward.
Factor 2 x 2 – 5 x – 12. Given equation y=ax^3 + bx^2 The solution (it's given after the exercise) is :. The graphs of quadratic functions are parabolas;.
So solving ax 2 + bx + c = 0 for x means, among other things, that you are trying to find x-intercepts.Since there were two solutions for x 2 + 3x – 4 = 0, there must then be two x-intercepts on the graph.Graphing, we get the curve below:. 16a + 4b = 8 (you can reduce this one to 2a + b = 2 by dividing both sides by 4) Can you solve the system of linear equations to find a and b?. In mathematical geometry, a parabola is a part of the conic.
The graph of the equation y =ax^2 + bx + c, where a, b, and c are constants, is a parabola with axis of symmetry x = -3. You can use either the substitution or elimination method. Suppose C < Da What Is The Change In Y'Constrained Due To A 3% Increase In C = 8?.
Solve for x y=ax^2+bx+c Rewrite the equationas. The graph passes through (4,0), hence, 0 = k(4 - 5)^2 - 3 and 0 = k - 3. Add '-1by' to each side of the equation.
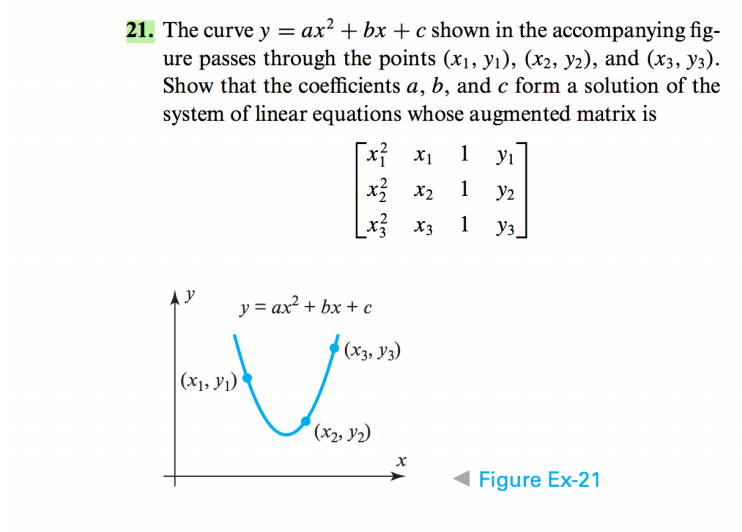
The curve y = ax 2 + bx + c passes through the point (2, 12) and is tangent to the line y - 4x at the origin. The graph of y = ax^2 + bx + c A nonlinear function that can be written on the standard form a x 2 + b x + c, w h e r e a ≠ 0 is called a quadratic function. Y = a(x 2 - 2xh + h 2) + k.
Recall that if there are solutions, they satisfy the quadratic formula. With just two of the parabola's points, its vertex and one other, you can find a parabolic equation's vertex and standard forms and write the parabola algebraically. O a -4,50.c1 O a = 1, b = 4,c=0 a = 2, b = 0,c=0 O a = 0,b=1,c=4.
Asked by Anonymous on March 5, 10;. Solution for x^2+y^2+ax+by+c=0 equation:. X is the independent variable, and y is the dependent variable.Quadratics are also called second degree polynomials because the highest exponent is 2.
4a + 2b = 0. For the first positions, find two factors whose product is 2 x 2.For the last positions, find two factors whose product is –12. Decide the direction of the paraola:.
The of an equation are equal to the of the function. Graph of y = ax 2 + bx + c, where a and the discriminant b 2 − 4ac are positive, with. At (1,4) the function is 4= a+b, and the.
8 = a4 2 + b4 = 16a + 4b. √b is the principle square root. Make room on the left-hand side, and put a copy of "a" in front of this space.
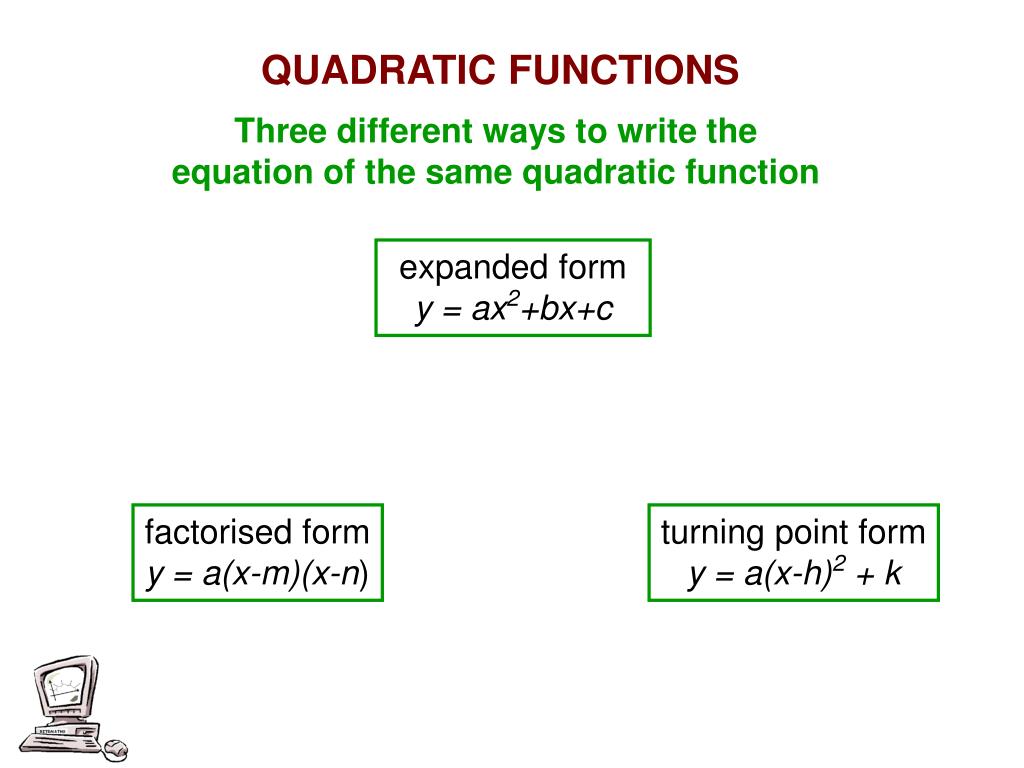
Y = a(x - h) 2 + k. $y=ax^2+bx$ with $a≠0$ by factoring, and use that to get a formula for the axis of symmetry of any equation in that form. This module aims to prepare the Form five students for the SPM examination and also for the Form four students to reinforce as well as to enable them to master the selected topics.
Try out your expected parameters. We want to put it into vertex form:. Begin by writing two pairs of parentheses.
Don't just watch, practice makes perfect. Study this pattern for multiplying two binomials:. Y = ax 2 + bx + c:.
Algebra -> Real-numbers-> SOLUTION:. Hence, your parabola is y = k(x - 5)^2 - 3. The parabola y=ax^2+bx+c has vertex (p,p) and y-intercept (0,-p), where p≠ 0.
36 is the value for 'c' that we found to make the right hand side a perfect square trinomial. In this section, you will add, subtract, multiply, and graph quadratics. Given that mathy=ax+bx^2/math math\frac{dy}{dx}=y’=a+2bx/math math\frac{d^2y}{dx^2}=y’’=2b/math then mathy=x\,y’-\frac{1}{2}x^2 y”/math.
Find a and b if the graph of y=ax^2 + bx^3 is symmetric with respect to (a) the y-axis and (b) the origin?. Estimate the free parameters. Please Calculate To 2 Decimal Places.
Use graphing to solve quadratic equations. Label a, b, and c. So given a set of 3 points (xy-plane), such as (40,30) (60,28) (,25) i have to find the equation of the parabola.
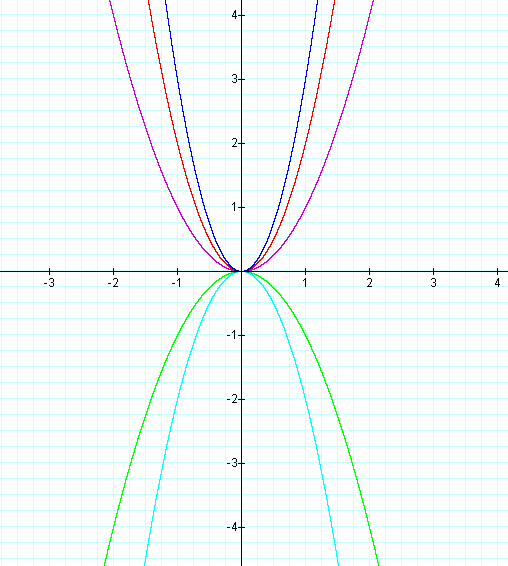
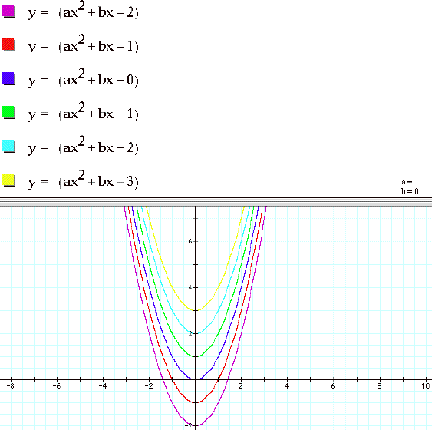
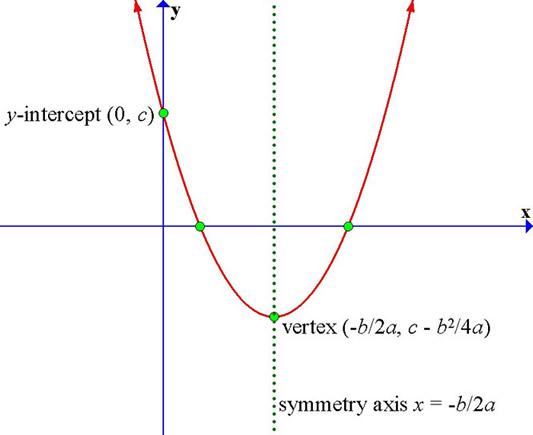
Y – c = ax 2 + bx:. Roots and y-intercept in red;. The standard format of a quadratic equation is y = ax 2 + bx + c;.
Get 1:1 help now from expert Calculus tutors Solve it with our calculus problem solver and calculator. There are four given clues 1) the y-intercept is (0,6) 2) the curve goes throuth (4,5) 3) the curve has aturning point at (2,3) 4) the line of symmetry is x=1 a)is it not possible for all four clues. Use the quadratic formulato find the solutions.
{\displaystyle y=ax^ {2}+bx+c,} which is a parabola. Max Y -ax2 + Bx S.t. Y'= 2ax+b, y' (1)=9 since y= 9x-5 is the tangent line.
Ok, simple question, having trouble understanding this in school. What is the other factor?. Write the vertex form of a quadratic function.
Find a, b, and c. Parabola and Linear Equations:. C > X Suppose A = 19 And B = 190.
Y = ax 2 + bx + c In this exercise, we will be exploring parabolic graphs of the form y = ax 2 + bx + c, where a, b, and c are rational numbers. A number b such that a^2 = b. Graph y = -x2 - Problem 7:.
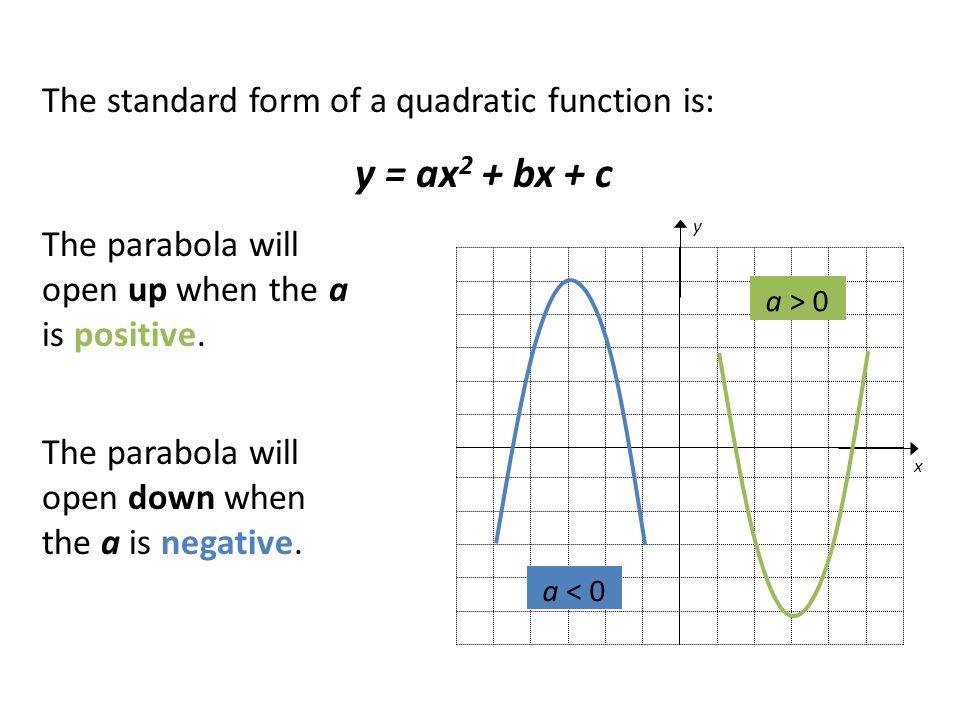
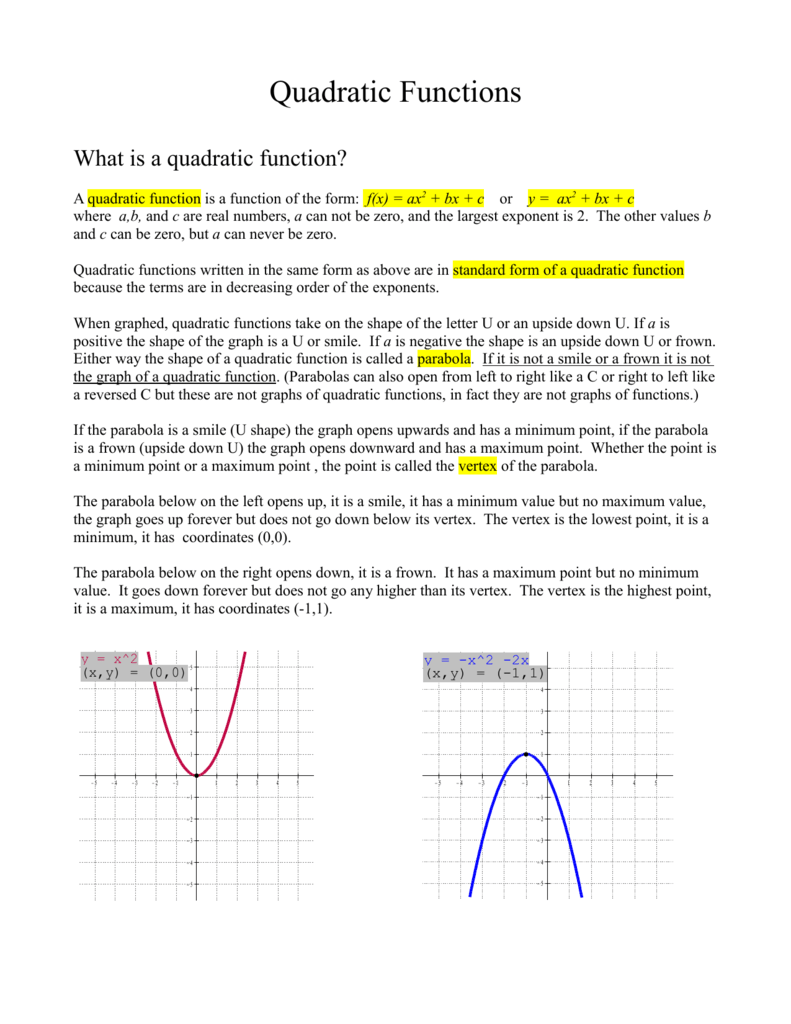
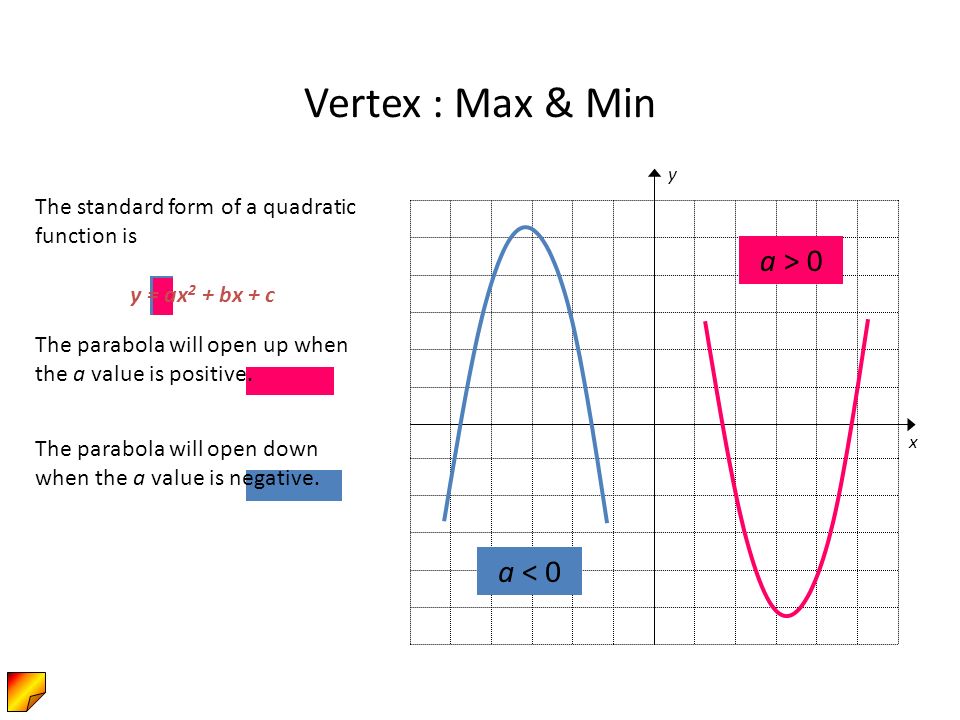
They tend to look like a smile or a frown. A, b, c are constants;. The graph of a quadratic function is a parabola, a U-shaped curve that opens up or down.
Graph y = 2x Problem 3:. Find the parabola {eq}y = ax^2 + bx + c {/eq} passing through the points ( -1, 9), (1, -1), and (2, 3). Ax + by + c + x 2 + y 2 = 0 Solving ax + by + c + x 2 + y 2 = 0 Solving for variable 'a'.
Explorations of the graph. I need help setting up the problem and how to find the answers. Graph y = ½x Problem 4:.
Hence, k = 3. Every parabola has an axis of symmetry which is the line that divides the graph into two perfect halves. Ax + by + -1by + c + x 2 + y 2.
What is the solution set of the related equation 0 = ax 2 + bx + c?. Hi there, I've been trying to do a sum but I'm not succeeding in solving the sum no matter how much I try. In algebra, quadratic functions are any form of the equation y = ax 2 + bx + c, where a is not equal to 0, which can be used to solve complex math equations that attempt to evaluate missing factors in the equation by plotting them on a u-shaped figure called a parabola.
Find the parabola with equation y = ax^2 + bx whose tangent line at (1, 4) has equation y = 9x − 5?. Chapter 4 - QUADRATICS INTRODUCTION TO QUADRATICS Objectives. Graph y = x2 - 40 Problem 6:.
Graph y = x Problem 2:. The quadratic function y = ax 2 + bx + c is related to the equation ax 2 + bx + c = 0 by letting y equal zero. If the curve fit has the form y = Ax 2 + Bx + C, and your model for the trajectory of the object has the form y (t) = y 0 + v 0 + 1 2 gt 2, determine what the values of A, B, C and x are in terms of the quantities y 0, v 0, g and t.
0 = a2 2 + b2 = 4a + 2b. Get more help from Chegg. You can put this solution on YOUR website!.
We will graph this by first findingthe direction it opens up, the y intercept, the vertexand the axis of symmetry. The graph of y = ax^2 + bx + c. A) to be symmetric wrt the y-axis, that means that y(x) = y(-x).
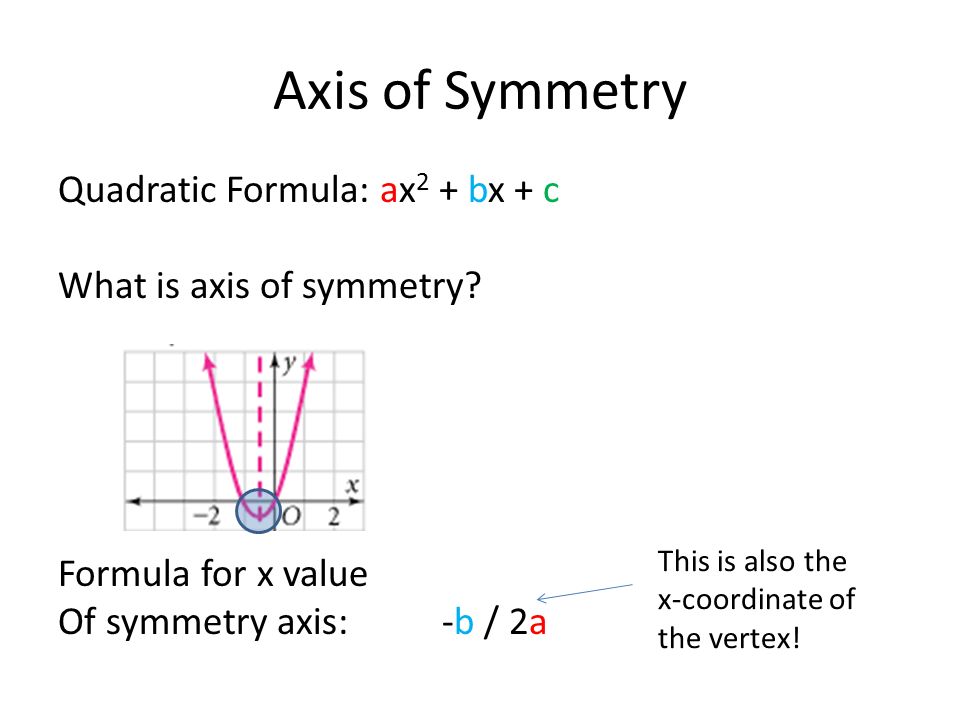
Suppose you have ax 2 + bx + c = y, and you are told to plug zero in for y.The corresponding x-values are the x-intercepts of the graph. The expression under the radical sign. Where a, b and c are real numbers, and a ≠ 0.
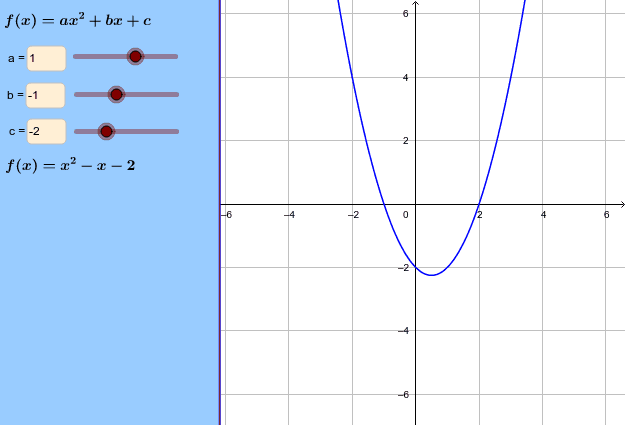
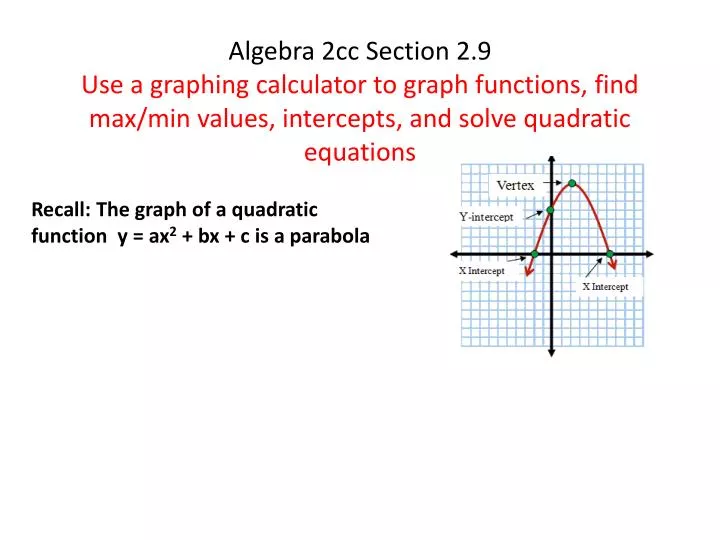
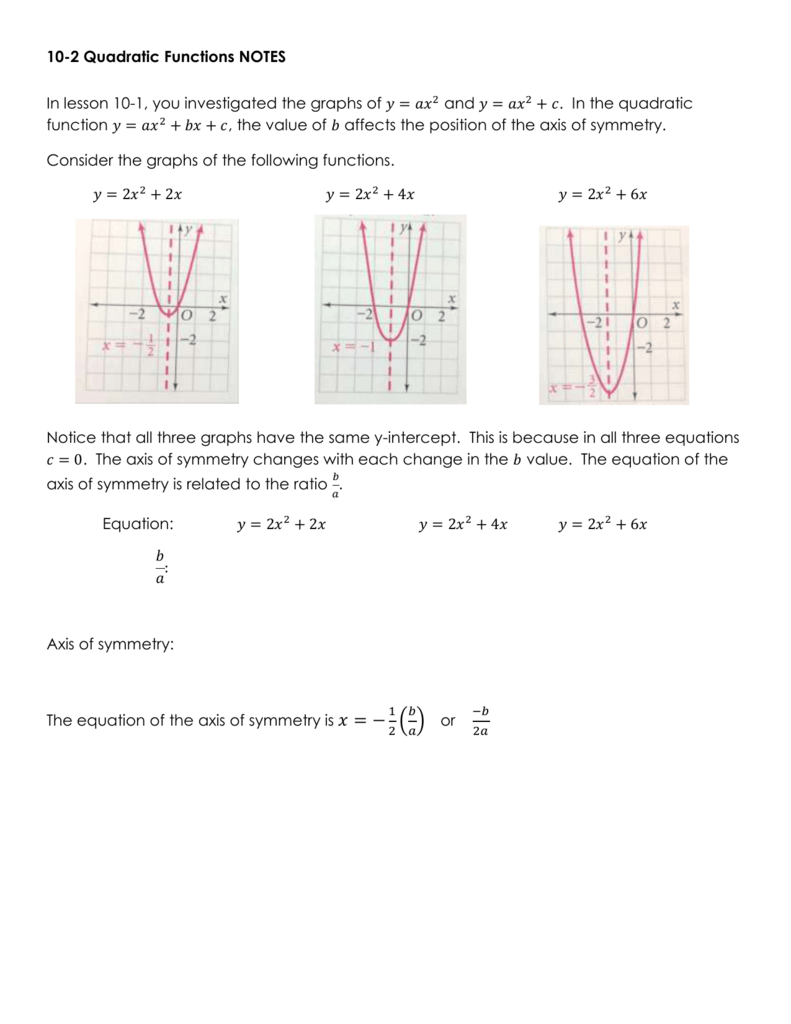
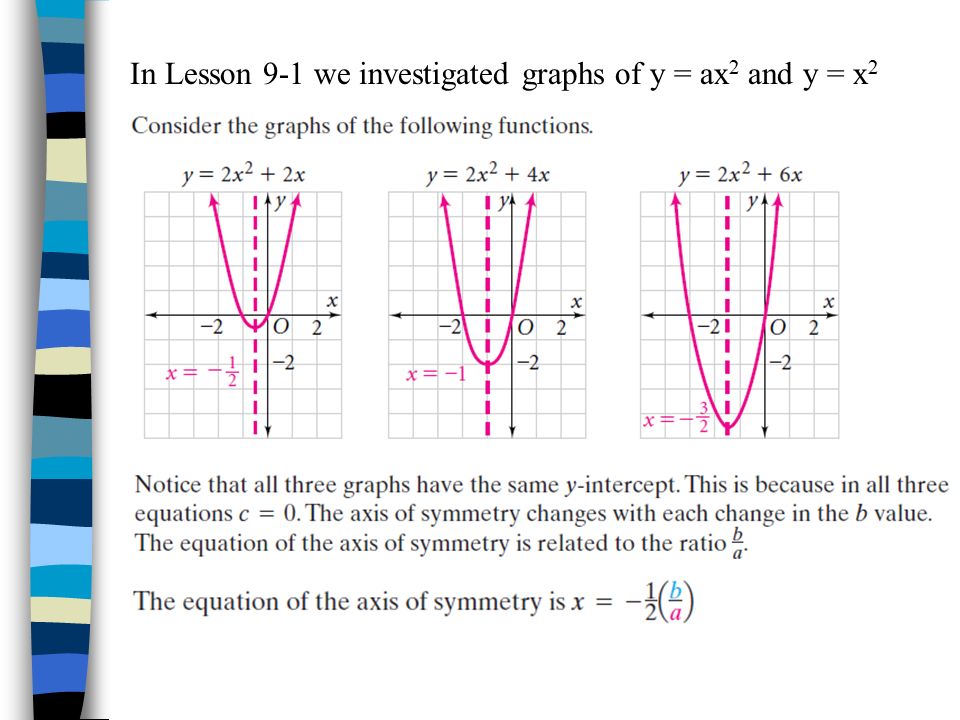
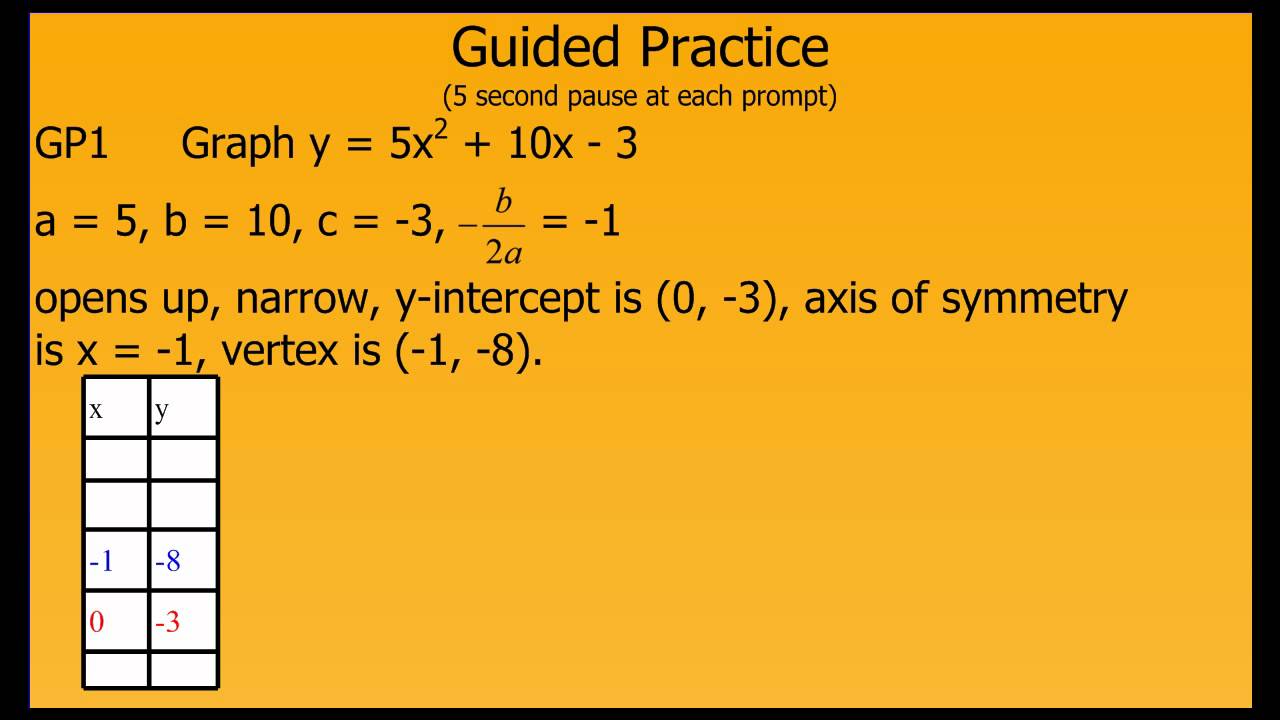
Y = ax 2 + bx + c. SummaryIn this presentation we are learning how to graphy = ax2 + bx + c. In particular, we will examine what happens to the graph as we fix 2 of the values for a, b, or c, and vary the third.
By Kristina Dunbar, UGA. Vertex and axis of symmetry in blue;. Move the loose number over to the other side.
Graphing y = ax^2 + c 1. The parabola is rotated 180° about its vertex (orange). If a < 0 (negative) then the parabola opens downward.
Free quadratic equation calculator - Solve quadratic equations using factoring, complete the square and the quadratic formula step-by-step. Graph y = -x Problem 5:. We have to form a differential equation by eliminating arbituary values from the given equation.
Our equation is in standard form to begin with:. Move to the left side of the equationby subtracting it from both sides. In this particular example, the norm of the residual is zero, and an exact solution is obtained, although rcond is small.
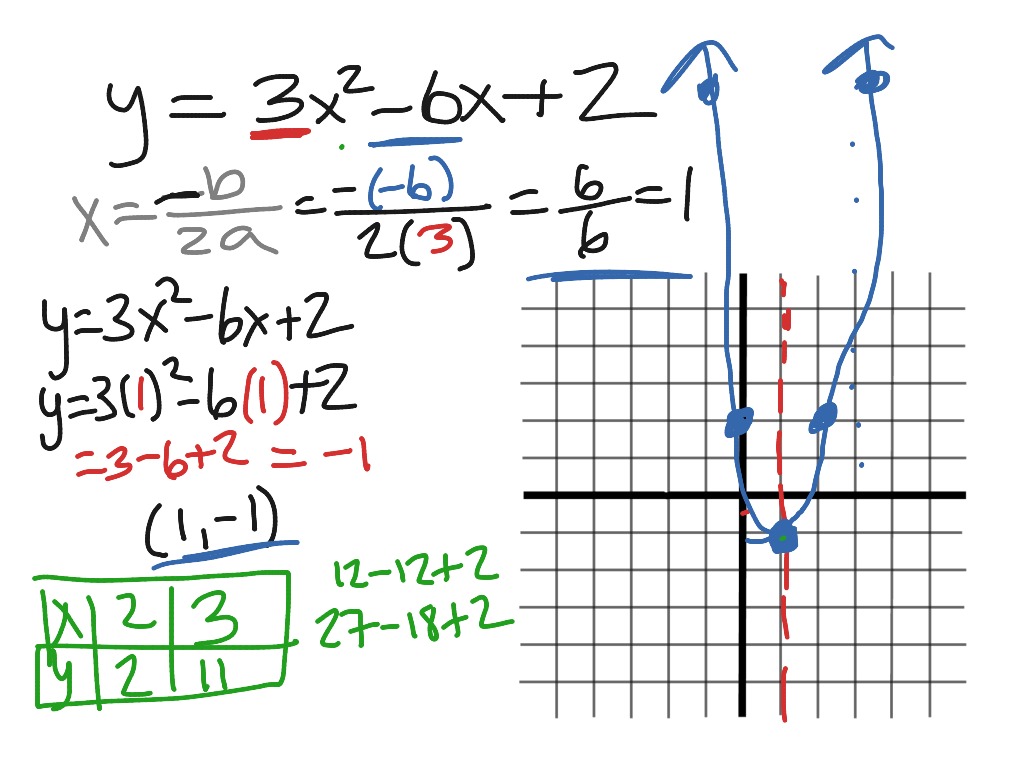
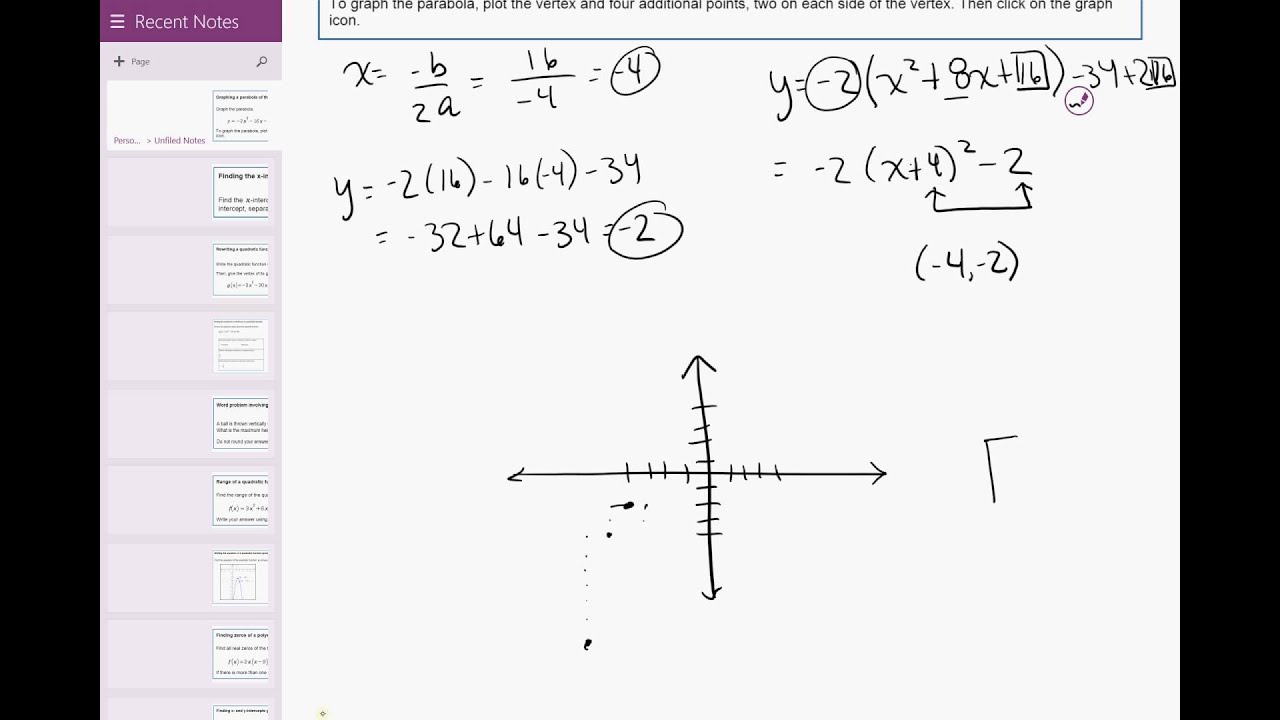
A quadratic y = ax^2 + bx + c crosses the x-axis when the equation 0 = ax^2 + bx + c has at least one solution. A is the coefficient of the x^2 term b is the coefficient of the x term c is the constant term they are used in equations to find the roots and in equations to find the minimum / maximum point of a quadratic equation and in equations to find the slope and y-intercept of a straight line, among other uses that I am probably not totally aware of. Y=ax 2 + bx.
Plug in the two given points, (2,0) and (4,8):. The axis of symmetry of the parabola determined by the function y = ax 2 + bx + c is the line that. The graph of y = ax^2 + bx + c is a parabola that opens up and has a vertex at (-2, 5).
A function of the form y = ax^2 + bx + c, where a ≠ 0. Simplifying x 2 + y 2 + ax + by + c = 0 Reorder the terms:. Why do you think the x-intercepts are called zeros?.
We can convert to vertex form by completing the square on the right hand side;. Y= 3 y= ax^2 + b In the system of equations above, a and b are constants.For which of the following values of a and b does the system of equations have exactly two real solution Log On. Visualisation of the complex roots of y = ax 2 + bx + c:.
Using Vertex Form to Derive Standard Form. Factor out whatever is multiplied on the squared term. When rcond is between 0 and eps, MATLAB® issues a nearly singular warning, but proceeds with the calculation.When working with ill-conditioned matrices, an unreliable solution can result even though the residual (b-A*x) is relatively small.
We have split it up into three parts:. To find the unknown. We have over 1850 practice questions in Algebra for you to master.
Formula y = ax2 + bx + c 4. The equation y = ax 2 - 2axh + ah 2 + k is a quadratic function in standard form with. Graph linear equation of the form y=ax.
Focus and directrix in pink;. A free graphing calculator - graph function, examine intersection points, find maximum and minimum and much more. We want to factor $ax^2+bx$.
On this page, we will practice drawing the axis on a graph, learning the formula, stating the equation of the axis of symmetry when we know the parabola's equation. It also serves as a guidance for effective acquisation of the various. Y = ax 2 - 2ahx + ah 2 + k.
Exploring Parabolas Y Ax 2 Bx C
Q Tbn 3aand9gcrj4zefjn32tclwxwszidmmbulqtnieycx Jyr6u1jhn9b002k8 Usqp Cau
Standard Form Y Ax2 Bx C Factored Form Y A X S X T
Yax2+bx のギャラリー
10 1 Quadratic Graphs Ppt Download
The Graph Of Y Ax 2 Bx C Geogebra

Kwadratische Vergelijkingen Oplossen Y Ax2 Bx C Video 2 Wiskunde Tv Youtube
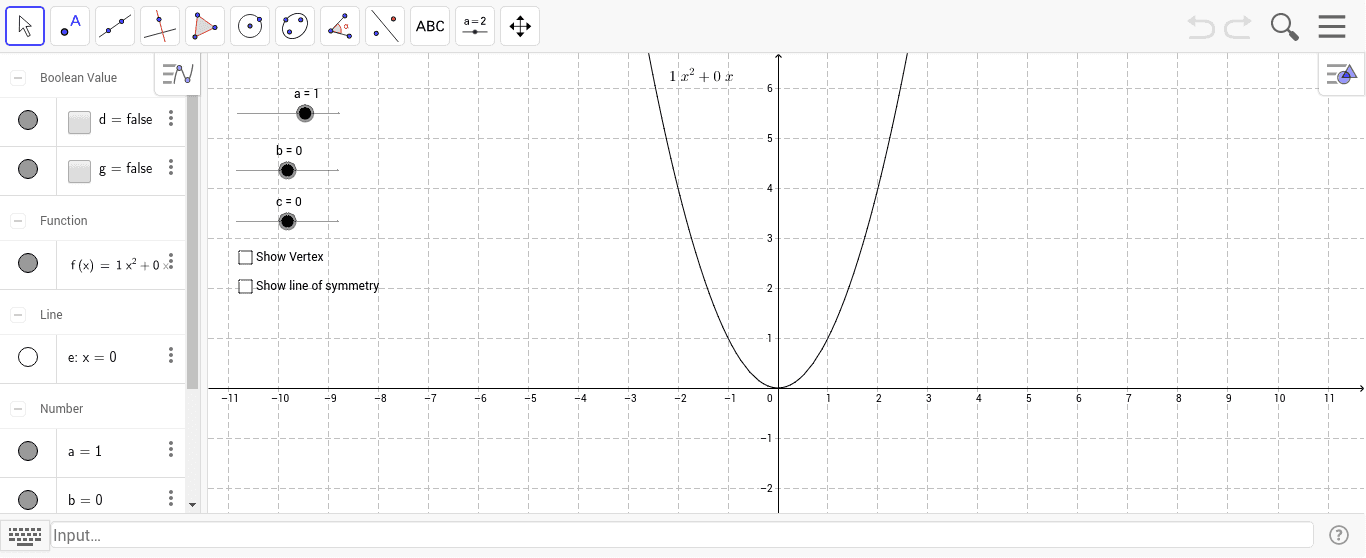
Quadratic In Standard Form Y Ax 2 Bx C Alter The Coef Geogebra

Solved Use The Graph Of Y Ax2 Bx C To Solve A Quadr Chegg Com

Find Differential Equation Of Family Of Curves Y Ax B X Brainly In

Sat Math Grid Ins Question 97 Answer And Explanation Cracksat Net
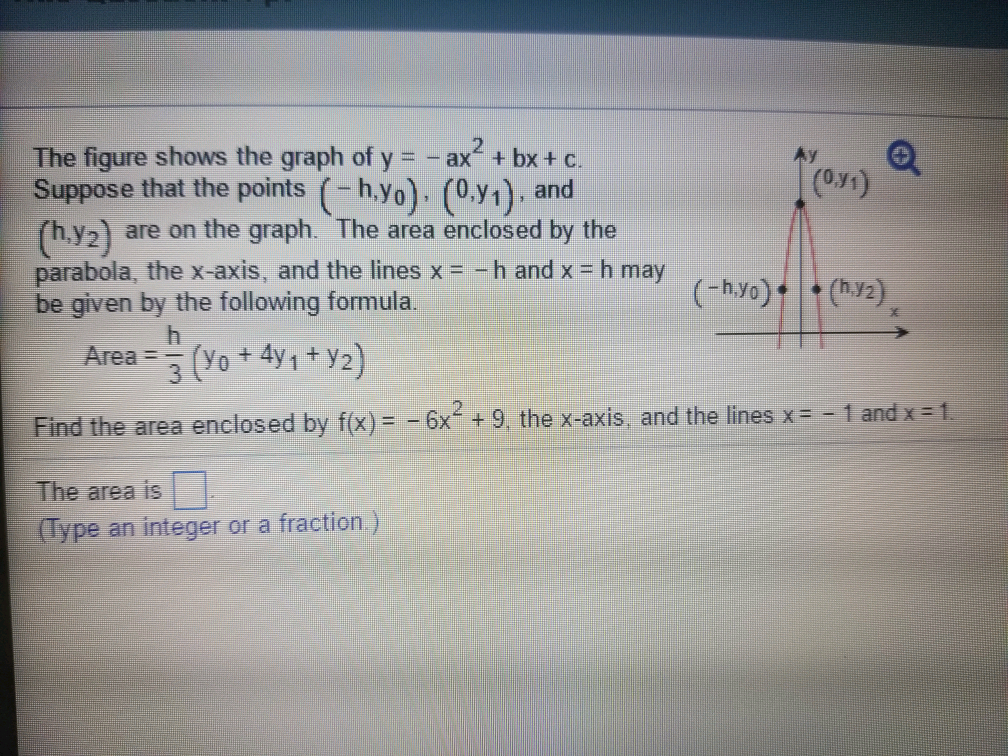
Solved The Figure Shows The Graph Of Y Ax 2 Bx C Chegg Com
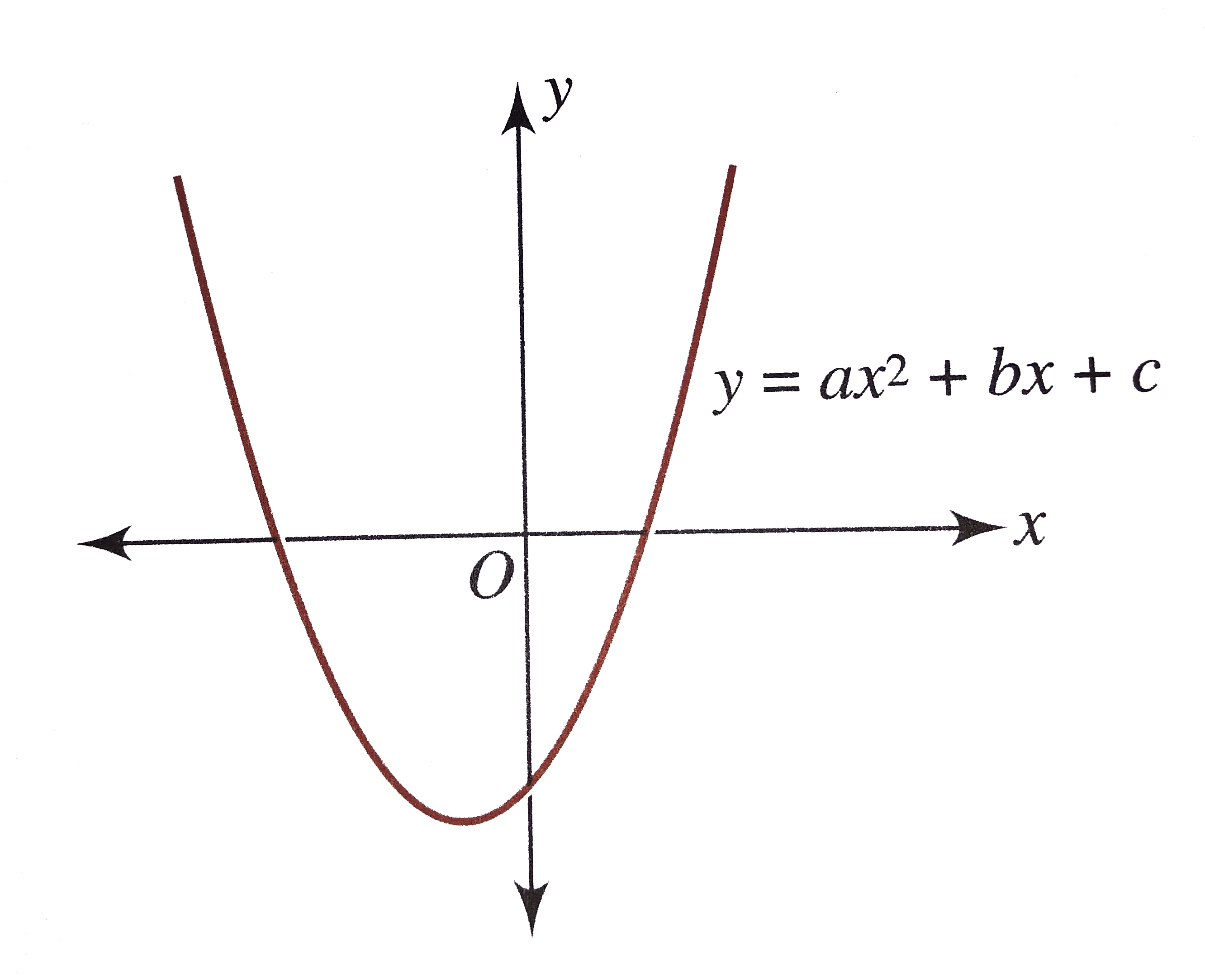
For Following Graphs Of Y Ax 2 Bx C With A B C C R Commen

Solve Graphing Quadratic Functions

To Investigate The Effect Of The Constants A B And C On The Graph Y Ax2 Bx C Gcse Maths Marked By Teachers Com
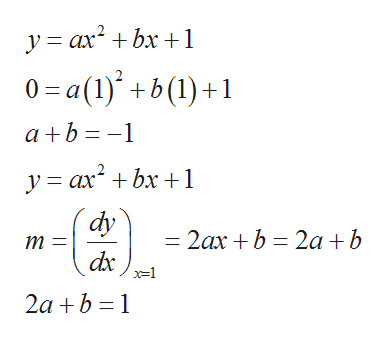
Answered Find An Equation Of The Parabola Y Bartleby

View Question A Parabola With Equation Y Ax 2 Bx C Has A Vertical Line Of Symmetry At X 1 And Goes Through The Two Points 1 3 And 2 2 The Quadratic
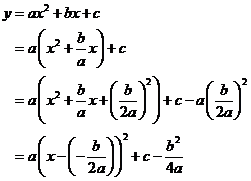
Assignment 2 Investigating The Relationship Between The Two Standard Forms Of The Graph Of A Parabola
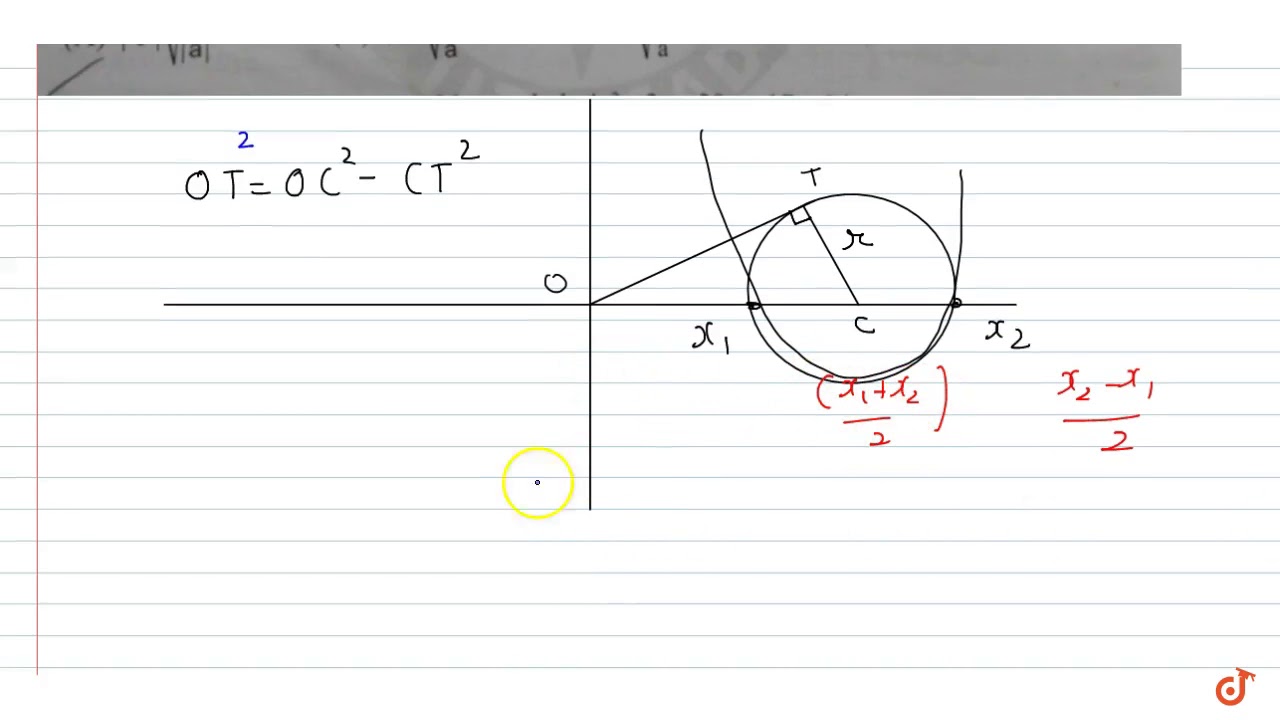
A Parabola Y Ax 2 Bx C Ac Gt 0 Crosses The X Axis At A And B A Variable Circle Is Dra Youtube

How To Complete The Square Of Quadratic Equation Of Y Ax 2 Bx C By Mthokozisi Issuu

Unique Quadratic Equation In The Form Y Ax 2 Bx C
Suppose A Parabola Y Ax 2 Bx C Has Two X Intercepts One Pos
Quadratic Function Wikipedia

To Investigate The Effect Of The Constants A B And C On The Graph Y Ax2 Bx C Gcse Maths Marked By Teachers Com
Q Tbn 3aand9gcq10yevvo7obji9orjkaelaoicwfrfgmucrgbvhjvjuqt162v0l Usqp Cau
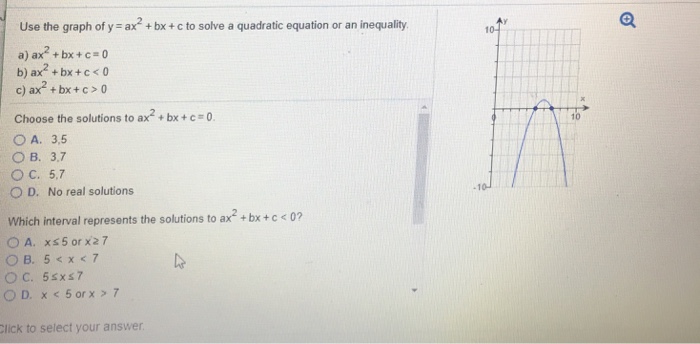
Solved Use The Graph Of Y Ax 2 Bx C To Solve A Quad Chegg Com
Ppt Recall The Graph Of A Quadratic Function Y Ax 2 Bx C Is A Parabola Powerpoint Presentation Id
Solved The Curve Y Ax 2 Bx C Shown In The Accompany Chegg Com
Finding The Inverse Of Y Ax2 Bx C By Completing The Square Youtube
Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube
Graph Of A Quadratic Function

Math 140 Lecture 28 Y Ax2 Bx C University Of San Diego Pages 1 5 Text Version Anyflip
10 2 Graph Ax 2 Bx C Math Showme

The Expression Y Ax 2 Bx C Has Always The Same Sign As Of A If A 4ac Lt B 2 B 4ac Youtube
Show That Y Ax 2 Bx C A 0 Represents A Parabola And Find Its Vertex Focus Directrix And Latus Rectum Sarthaks Econnect Largest Online Education Community
Chapter 10 Section 2 Review Quadratic Function Y Ax 2 Bx Cy 2x 2 3x 1 Symmetry Ppt Download
15 04 Graphing A Parabola Of The Form Y Ax 2 Bx C Integer Coefficients Youtube
Graphing Quadratics
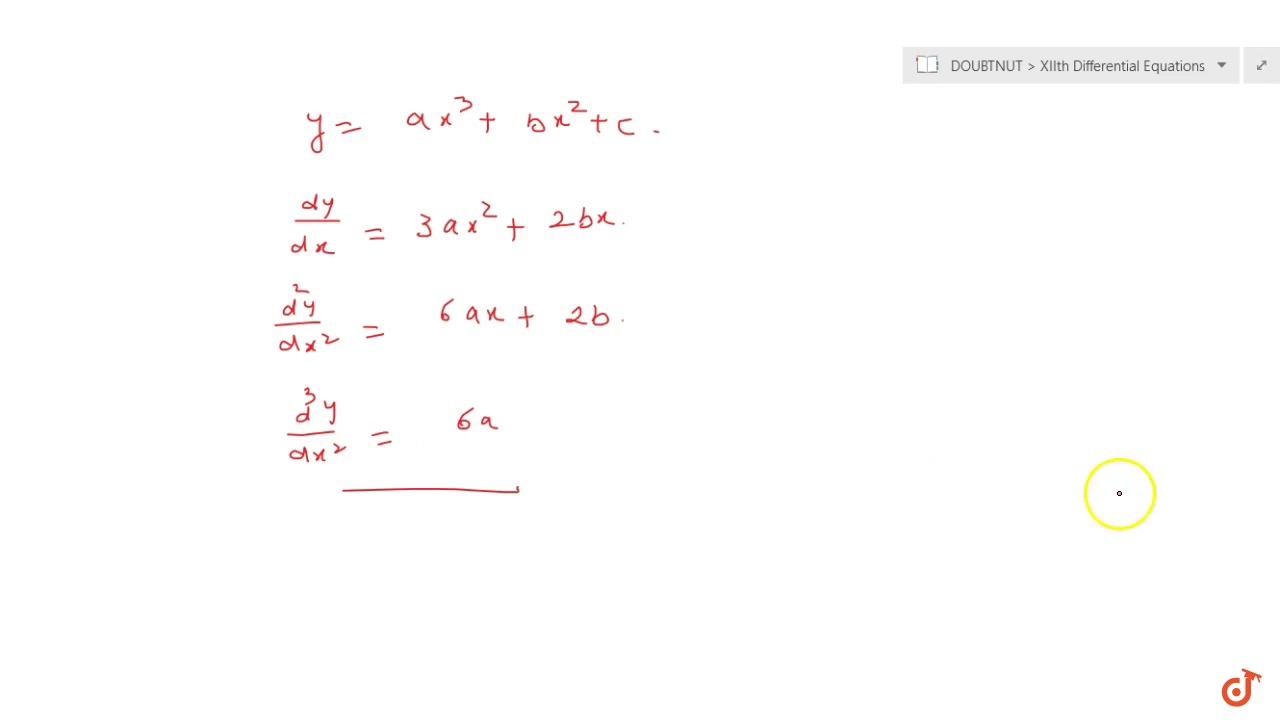
Show That Y A X 3 B X 2 C Is A Solution Of The Differential Equation D 3y Dx 3 6a Youtube
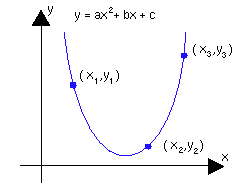
Linear Algebra Webnotes Class Assignments Chapter 1
Q Tbn 3aand9gcslv Mws2ur19dxxvsr04ksazybsceybissdje0bez9zpdcftj2 Usqp Cau
Quadratic Relations Y Ax 2 Bx C Between The A N B P And Download Scientific Diagram

The Graphs Of Y Ax 2 Bx C Are Given In Figure Identify The Signs Of A B And C In Each Of The Following
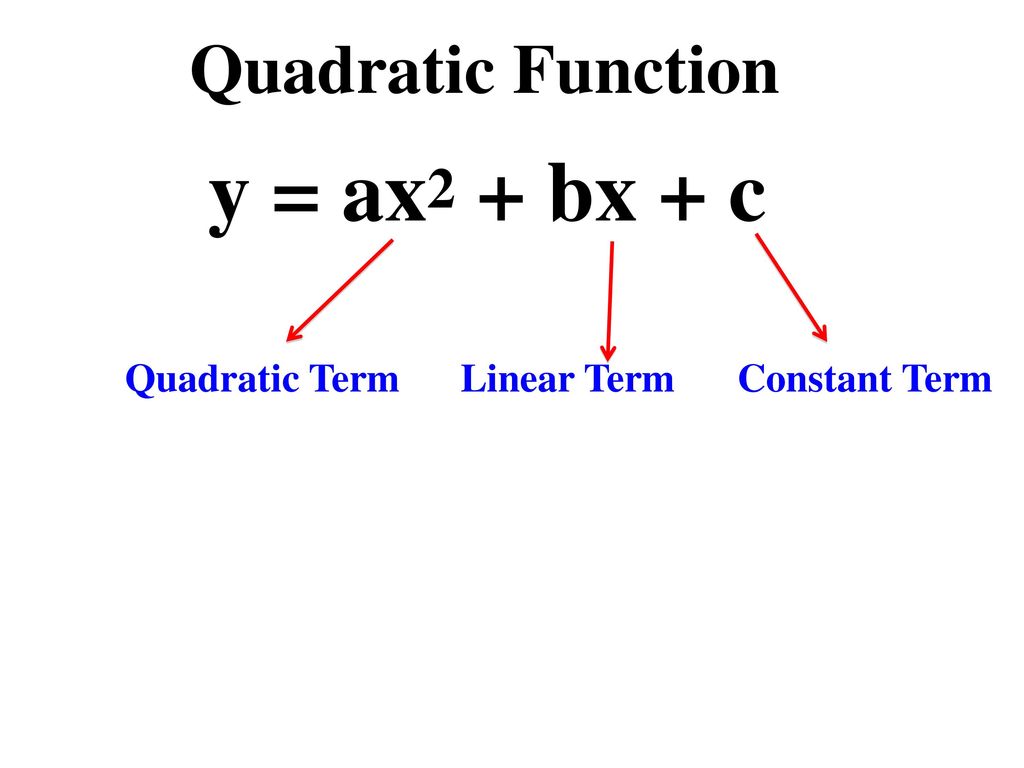
Y Ax2 Bx C Quadratic Function Quadratic Term Linear Term Ppt Download

Math 140 Lecture 28 Y Ax2 Bx C University Of San Diego Pages 1 5 Text Version Anyflip
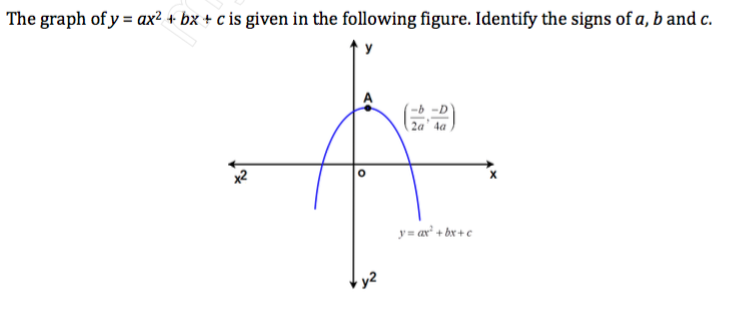
Solved The Graph Of Y Ax 2 Bx C Is Given In The Fol Chegg Com

Graphing A Parabola Of The Form Y Ax2 Bx C With Integer Coefficients Youtube
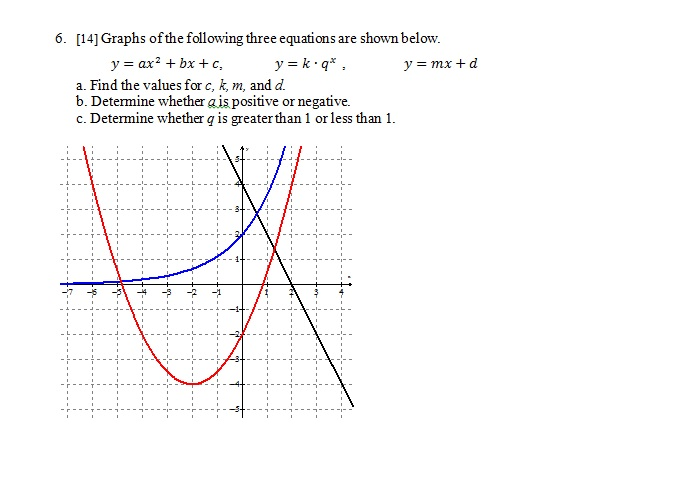
Solved Graphs Of The Following Three Equations Are Shown Chegg Com

For Problems 29 31 The Graph Of A Quadratic Function Y Ax 2 Bx C Is Shown Tell Whether The Brainly Com
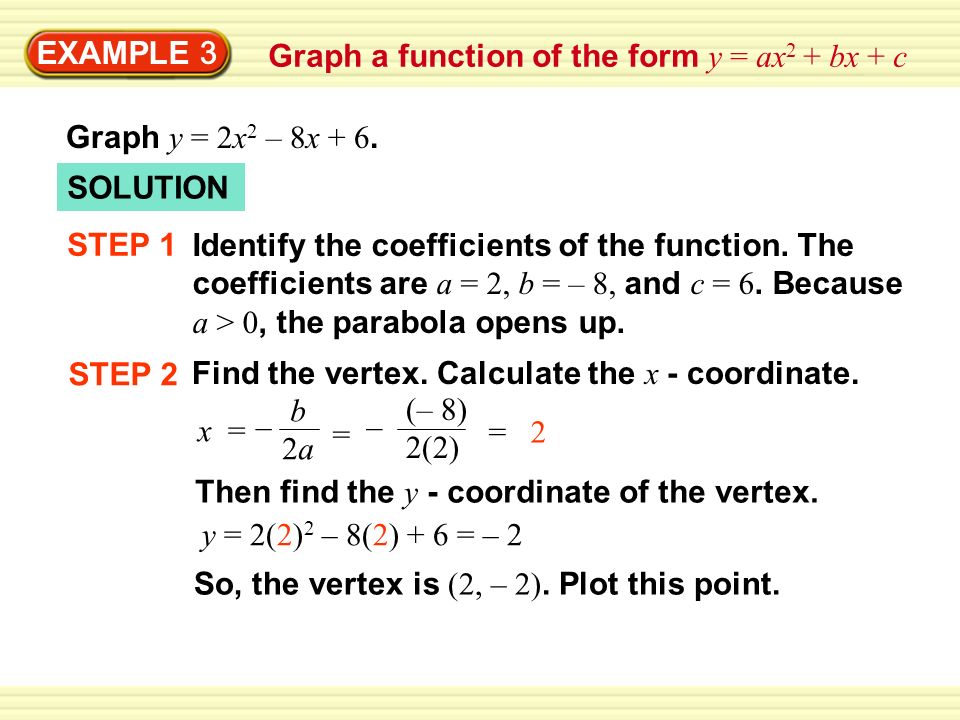
Example 3 Graph A Function Of The Form Y Ax 2 Bx C Graph Y 2x 2 8x 6 Solution Identify The Coefficients Of The Function The Coefficients Ppt Download

Consider The Graph Of Quadratic Polynomial Y Ax 2 Bx C As Shown Below Which Of The Following Is Are Correct

Graphing Y Ax 2 Bx C

Act Math Practice Question 1184 Answer And Explanation Crackact Com
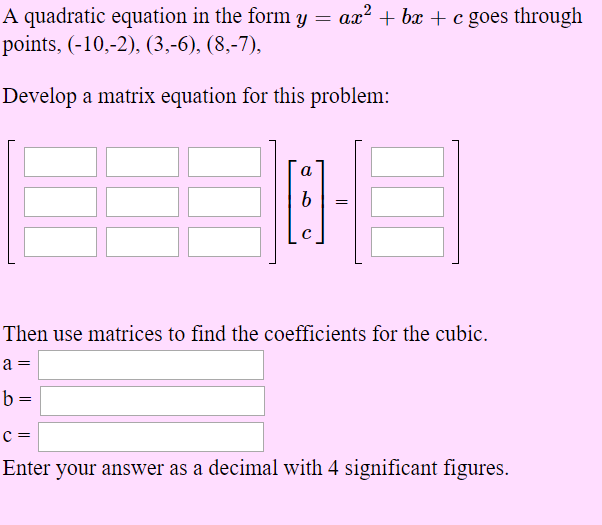
Solved A Quadratic Equation In The Form Y Ax 2 Bx C Chegg Com

A Curve Has The Equation Y Ax 3 Bx 2 Cx D The Curve Has A Point Of Inflection At 2 22 And The Equation Of The Tangent To The

Investigating Y Ax 2 Bx

To Investigate The Effect Of The Constants A B And C On The Graph Y Ax2 Bx C Gcse Maths Marked By Teachers Com
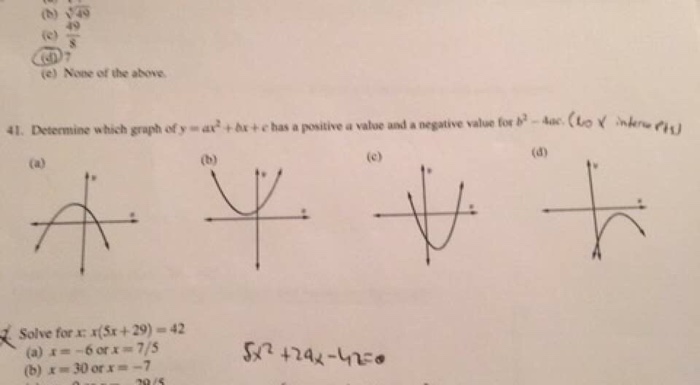
Solved Determine Which Graph Of Y Ax 2 Bx C Has A P Chegg Com
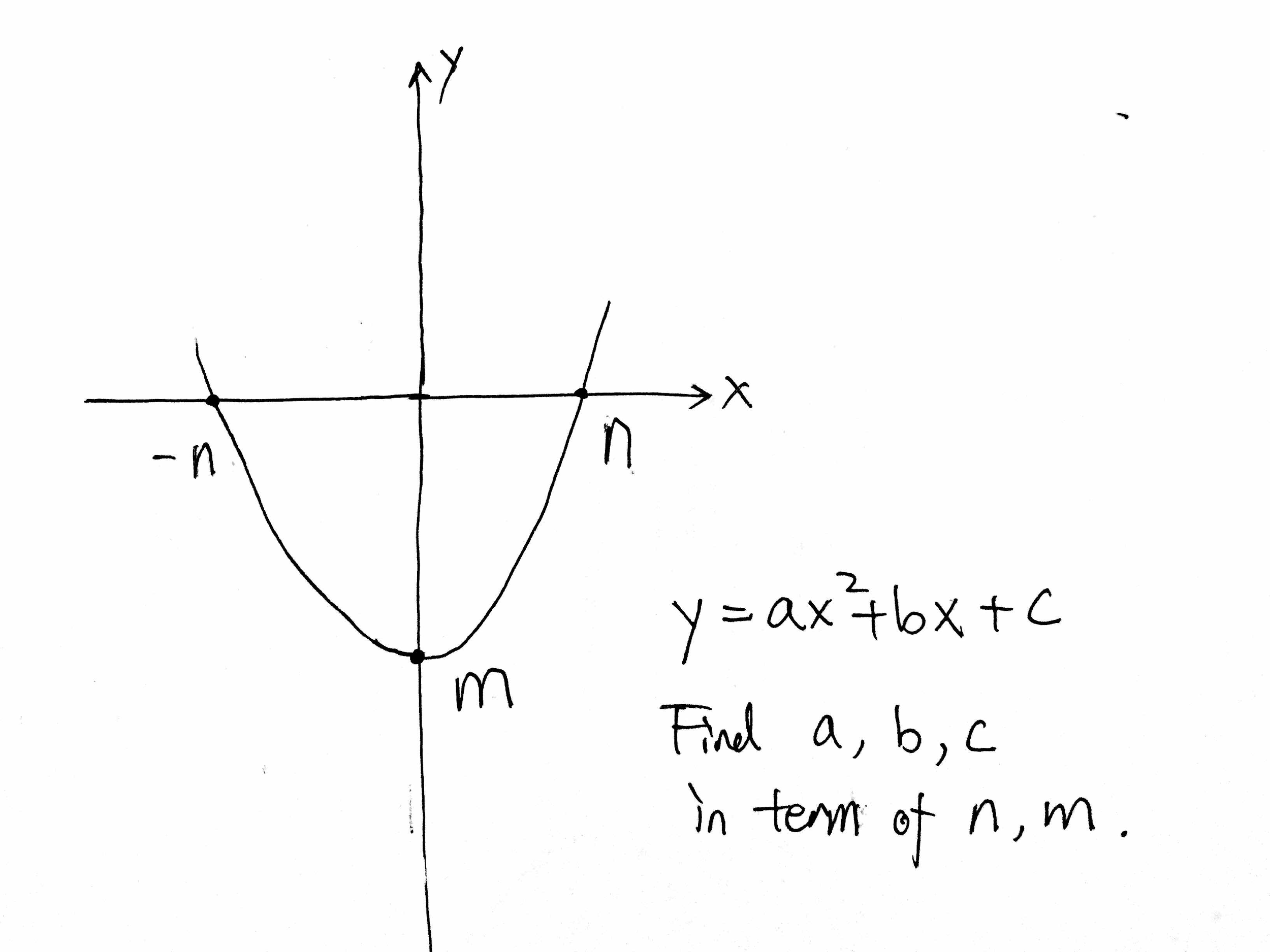
Solved Y Ax 2 Bx C Find A B C In Term Of N M Chegg Com
Equations And Graphs
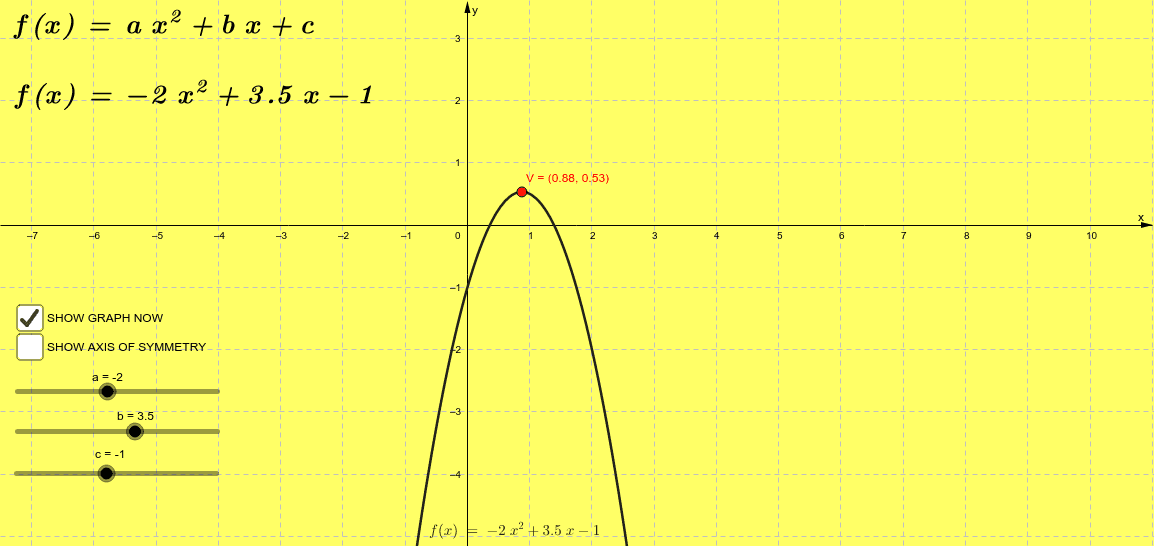
The Quadratic Function Y Ax2 Bx C Geogebra
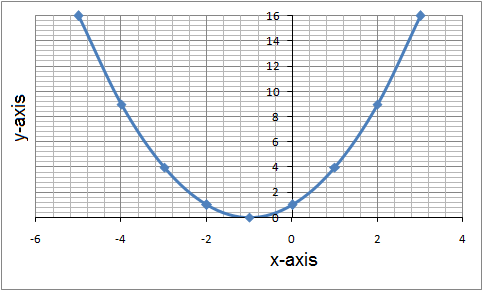
The Graph Of Y Ax 2 Bx C Algebra 1 Quadratic Equations Mathplanet

The Graph Of A Quadratic Function Y Ax 2 Bx C Is Shown Tell Whether The Discriminant Of Ax 2 Brainly Com
Lesson 9 2 Graphing Y Ax Bx C Objective To Graph Equations Of The Form F X Ax Bx C And Interpret These Graphs Ppt Download
Graphing Y Ax2 Bx C Youtube

Prostate Gland Black And The Defined Pz Below Y Ax 2 Bx C Download Scientific Diagram

Assignment 2
Quadratic Graph Example Y Ax C Expii

The Diagram Shows The Graph Of Y Ax 2 Bx C Then
Solution The Tangent To The Curve Y Ax 2 Bx 2 At 1 0 5 Is Parallel To The Normal To The Curve Y X 2 6x 4 At 2 4 Find The Value Of A And B

Refer The Picture Also Y Ax 2 Bx C Hd Png Download Transparent Png Image Pngitem

Solved A Form The Differential Equation From Y Ax 2 Chegg Com
If Y Ax 2 Bx C Represents A Parabola With Vertex A As Shown In The Figure And B 2 2 B 2ac Sarthaks Econnect Largest Online Education Community
Graphing Quadratic Functions Quadratic Functions Have The Form Y Ax 2 Bx C When We Graph Them They Make A Parabola Ppt Download

Math 140 Lecture 28 Y Ax2 Bx C University Of San Diego Pages 1 5 Text Version Anyflip
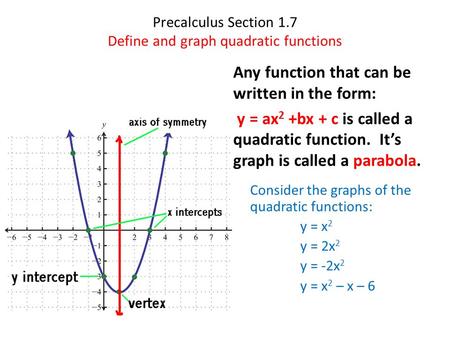
9 3 Graphing Y Ax Bx C 2 1a Y X 1 For 3 X 3 2 X Y B Does It Open Up Or Down C Identify The Axis Of Symmetry And Its Vertex Ppt Download
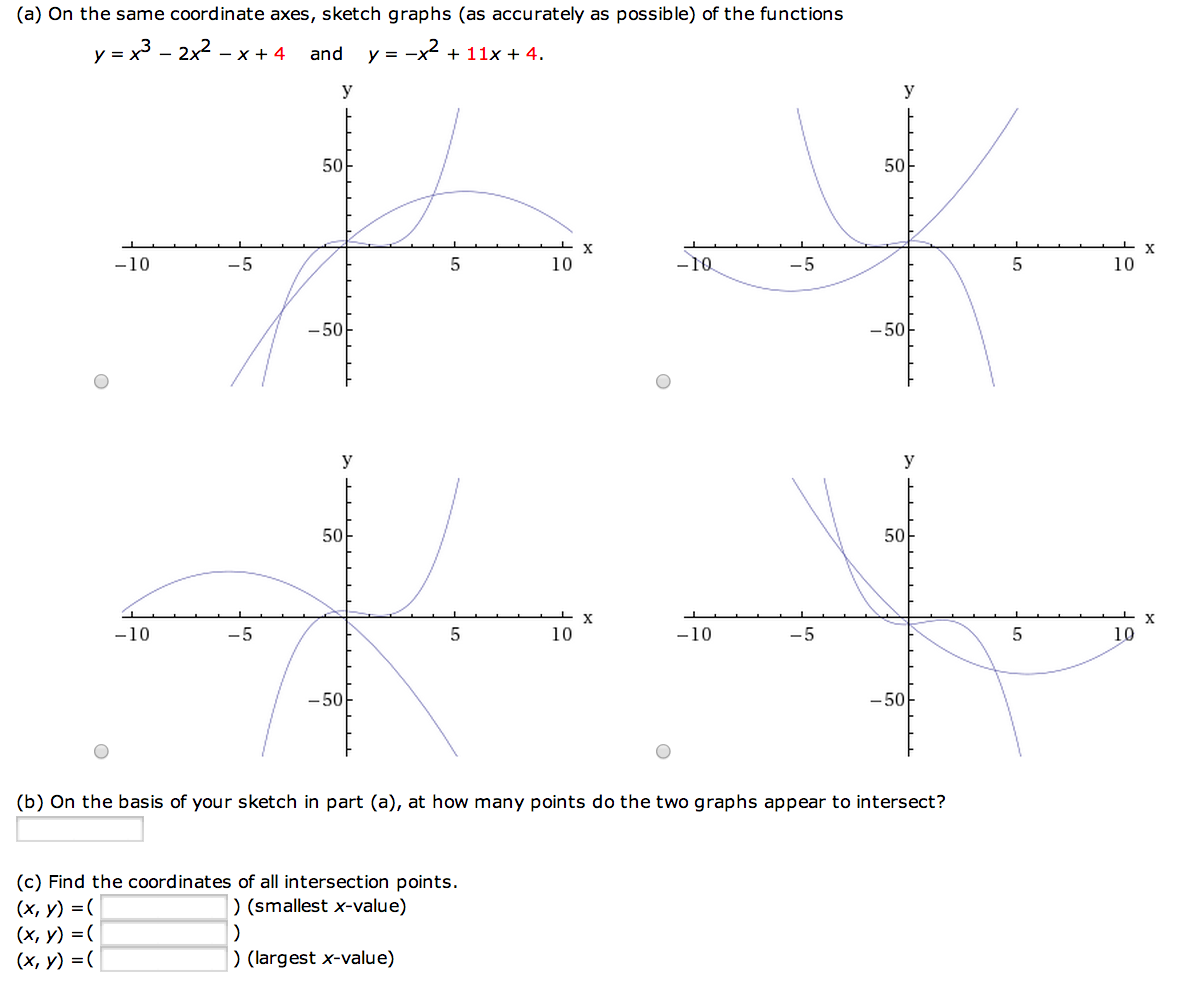
Solved Find A Parabola With Equation Y Ax2 Bx C Tha Chegg Com
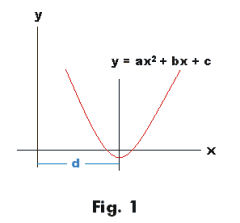
The Function F X Y And Associated Matrices E And E

Unique Quadratic Equation In The Form Y Ax 2 Bx C
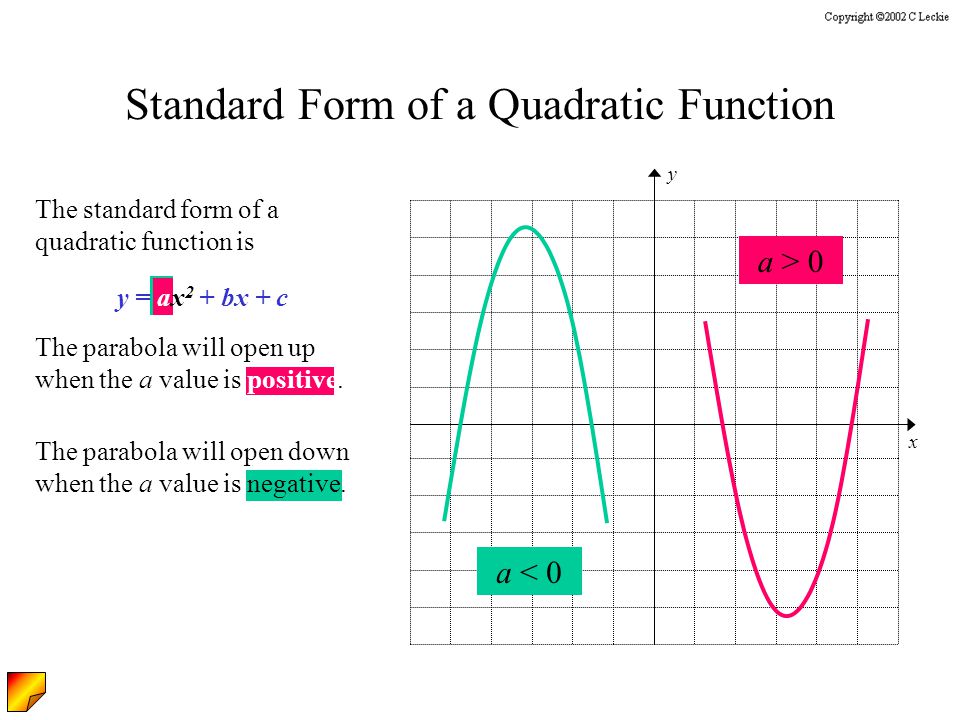
Lesson 10 2 Quadratic Functions And Their Graphs Y Ax 2 Bx C Ppt Download
Assignment 2
Ppt Standard Form Of Quadratic Function Y Ax 2 Bx C Powerpoint Presentation Id
Ppt Expanded Form Y Ax 2 Bx C Powerpoint Presentation Free Download Id 322
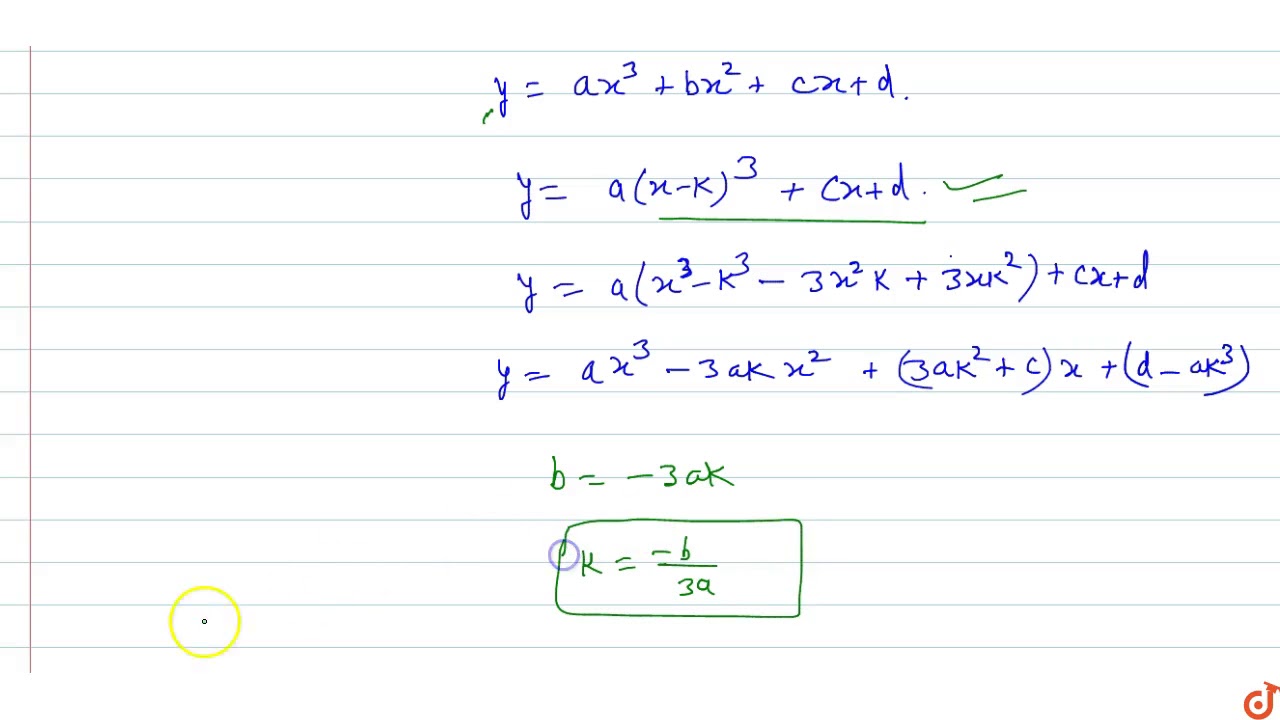
If The Graph Of Y Ax 3 Bx 2 Cx D Is Symmetric About The Line X K Then The Value Of A K Youtube

The Graph Of Y Ax 2 Bx C Is Shown Below Determine The Solution Set Of 0 Ax 2 Bx C 1 Brainly Com

Solution Discriminating Quadratics Underground Mathematics
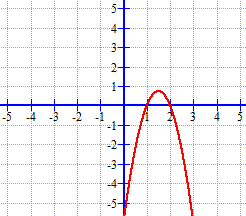
Braingenie Graphing Y Ax Sup 2 Sup Bx C Using A Table Of Values

How To Speak Y Ax 2 Bx C Englishteststore Facebook
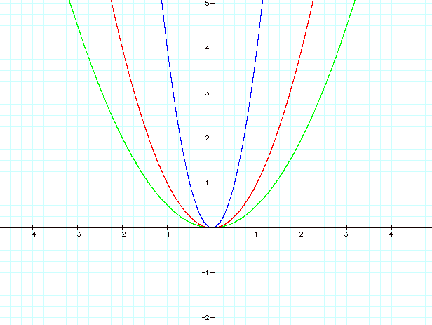
Examining The Parabola Assignment 2
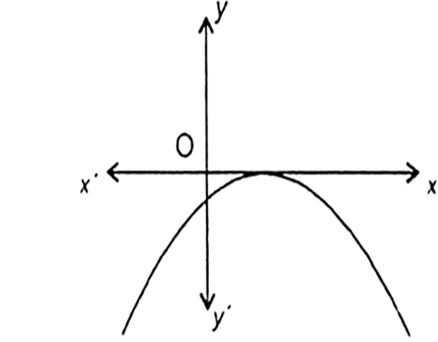
The Graph Of The Polynomial Y Ax2 Bx C Is Shown In Fig 2 2 Write The Value Of B2 4ac Y Ax2 Bx C From Mathematics Polynomials Class 10 Haryana Board English Medium
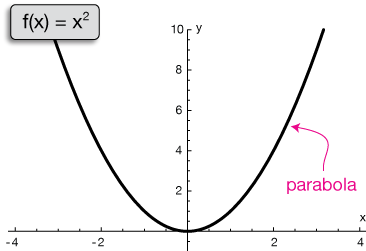
Quadratic Function Y Ax2 Bx C When A Is Sutori
Quadratic Functions
Assignment 2 Investigating The Relationship Between The Two Standard Forms Of The Graph Of A Parabola

6 2 Solve Quadratic Equations By Graphing
Graphing Quadratic Functions Y Ax 2 Bx C Graphing Quadratic Functions Today We Will Understand How The Coefficients Of A Quadratic Function Influence Ppt Download
If Y 2 Ax 2 Bx C Then Y 3 D 2y Dx 2 Is A A Constant Sarthaks Econnect Largest Online Education Community
Q Tbn 3aand9gcsnpfmyrsbee4qqbgpytnqudex6h1o5ppxcnfwrdthmlgplrmad Usqp Cau

The Graph Of Y Ax 2 Bx C Algebra 1 Quadratic Equations Mathplanet
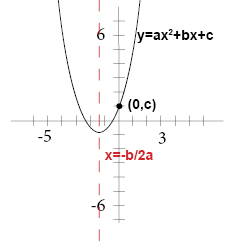
The Graph Of Y Ax 2 Bx C Algebra 1 Quadratic Equations Mathplanet

Graphing Y Ax 2 Bx C
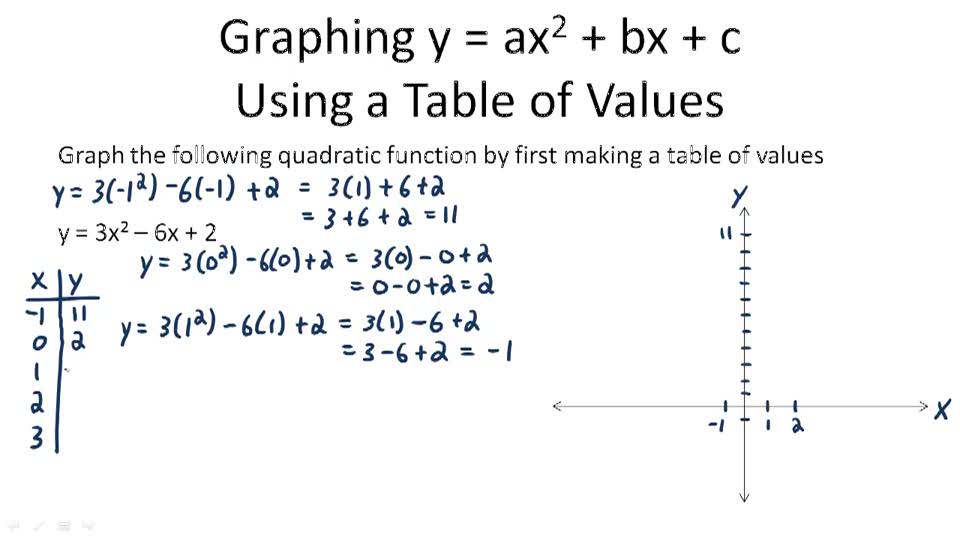
Graph Quadratic Functions And Equations Video Algebra Ck 12 Foundation
Solution Draw A Sketch Graph Of Y Ax 2 Bx C If A Lt 0 B Lt 0 C Lt 0 Discriminant 0

2 Graph Of Y Ax 2 Bx K Graph Of Y Mx K Download Scientific Diagram
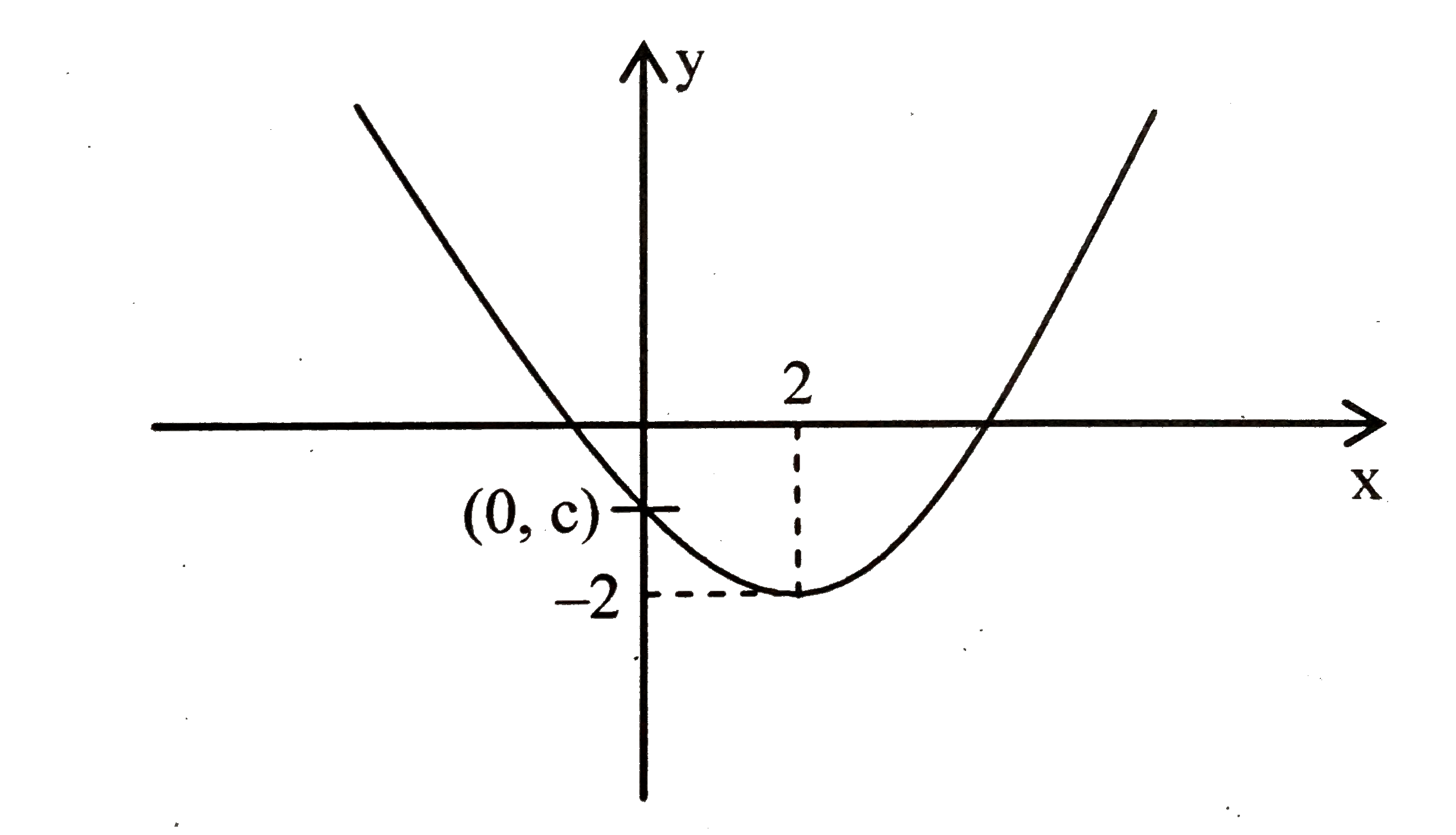
The Graph Of Y Ax 2 Bx C Has A Minimum At 5 3 And Passes Through 4 0 How Do I Find The Values Of A B And C Quora

Regression Analysis Of The Relationship Y Ax 2 Bx C Between Download Table




